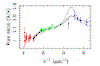
Old Q0957+561 data & software:
► Simple FORTRAN programs to apply the d2 test (accurate and robust time delay measurements)
► Photos
Advertising:
Introduction to GL
![]()
(i)
Brief historical Introduction
Surely, Newton's
Theory of Gravitation (1687) is one of the most important theories in
the history of science. It is not only able to describe the falling of an
apple, but also the formation of a galaxy. The equation of
gravitational force is one of the greatest conquests of Mankind:
When Newton published “Opticks” in
1704, he belived in the corpuscular nature of ligth, and he ensured that
it must exist a relation between ligth and matter, with the form of a gravitational
force ruled by equation [1].
In 1804 (two centuries ago), Soldner was the first
who calculated that, for small angles, the
Newtonian deflection of light by a massive object should be [SOL04]:
where M is the
mass of the deflecting object and R is the deflection impact parameter.
For a light ray grazing the Sun this gives a deflection angle
![]() (a scheme is shown in figure
1). This work was considered a theoretical curiosity and could not be
tested observationally, because of the lack of precision of telescopes in
1804.
(a scheme is shown in figure
1). This work was considered a theoretical curiosity and could not be
tested observationally, because of the lack of precision of telescopes in
1804.
|
|
|
figure 1: Newtonian
angle of deflection of light by the Sun. |
Although Newton’s Theory of Gravitation was acepted
by scientists along centuries, it was not able to explain several anomalies,
the most famous of these being the perihelion shift of Mercury. Classical
mechanics could explain the majority of the observed shift, but a residual
shift of
![]() per century could not be explained by the gravitational
effects of other planets.
per century could not be explained by the gravitational
effects of other planets.
During the
earlier part of this century, Einstein extended his Special Theory of Relativity
to generalized, or accelerating reference frames [EIN15]. From this study emerged a new description
of gravity which saw gravitational force as the curvature of a space-time,
the curvature being due to the presence of mass. General Relativity,
as this theory is known, not only explained the residual shift in Mercury's
perihelion, but also predicted other effects, including the bending of light
in a gravitational field. General Relativity predicts that the bending angle
for a light ray in the vicinity of a point mass to be [WEI72]:
precisely double the value
expected from Newtonian gravity (see equation [2]). With
this, light rays grazing the surface of the Sun are bent by an angle of
![]() (see figure 2). This
value is not twice that obtained by Soldner due to differing estimates for
the solar mass and radius at the time of calculation.
(see figure 2). This
value is not twice that obtained by Soldner due to differing estimates for
the solar mass and radius at the time of calculation.
In 1919, Eddington (on
Principe Island) and Crommlin (in Brazil), monitored the position of the
stelar background during the solar eclipse of the
![]() May, and they obtained a value for the deflecting angle of light by the sun
of
May, and they obtained a value for the deflecting angle of light by the sun
of
![]() and
and
![]() respectively, with quoted errors of
respectively, with quoted errors of
![]() . These results confirmed the Einstein’s prediction [EDI19].
. These results confirmed the Einstein’s prediction [EDI19].
In the following decades,
light deflection or Gravitational Lensing (GL) was only very rarely
the topic of a research paper: In 1924, Chwolson [CHW24] mentioned the idea of a “fictitous double
star” and the mirror-reversed nature of the secondary image. He also mentioned
the symmetric case of star exactly behind star, resulting in a circular image.
Einstein also reported in 1936 about the appearance of a “luminous circle”
(“Einstein Ring”, see figure 3) for perfect alignment
between source and lens [EIN36], and of two
magnified images for slightly displaced positions. Influenced by Einstein,
Fritz Zwicky [ZWI37] pointed out in 1937 that
galaxies (“extragalactic nebulae”) are much more likely to be gravitationally
lensed than stars and that one can use the gravitational lens effect as a
“natural telescope”.
In the 1960’s, a few
partly independent theoretical studies showed the usefulness of lensing for
astronomy. In particular, Sjur Refsdal derived the basic equations of Gravitational
Lens Theory and subsequently showed how the gravitational lens effect
can be used to determine Hubble's Constant by measuring the time delay between
two lensed images [REF64], [REF66].
When quasars were discovered
in the 1960’s, Barnothy [BAR65] was the first
to connect them with the gravitational lens effect.
In 1979 the whole field
received a real boost when the first double quasar (QSO 0957+561, see figure 4) was discovered and confirmed to be a real
gravitational lens by Walsh, Carswell & Weymann [WAL79].
![]()
Click on the arrow below to return to the previous page.